

A line is formed with an endless number of points. While lines have no definite beginning or end, they are displayed in our day-to-day lives with examples such as railway tracks or the freeway.Īns: The line is the path of one point moving. A line segment is only the part of a line.Īns: A line is a figure that is made when two points are connected with the minimum distance between them, and both the ends are extended to infinity. Here, the two points on the line are \(P\) and \(Q\). It is often defined as the shortest distance between any of the two points. A line is one-dimensional.Īns: The types of the lines are given below:Īns: In geometry, a line can be defined as a straight one-dimensional figure with no thickness and endlessly in both directions. So, it is made up of an endless number of points, and it is infinite and has no ends on both sides. A line is a type of geometric diagram that can move in both directions. Learn All the Concepts on Practical Geometry Frequently Asked QuestionsĪns: A line has a length, but it has no width. Finally, we have given solved examples along with a few FAQs. We glanced at the difference between the line and the line segment and examples followed by the importance of line. Then we have talked about types of lines that include horizontal, vertical, parallel, perpendicular, intersecting lines and transversal lines. In the given article, we have discussed the term line, then what a line segment is. This shows that the sum of the interior angles on the same side of the transversal \(CE\) is \(180°\), i.e., they are supplementary: Thus, lines \(AB\) and \(CD\) are intersected by the line \(BC\) such that \(∠ABC = ∠BCD\), i.e., the alternate angles are similar. Solved Examples– LinesĪns: Through \(O\) draw, the line \(l\) parallel to both the lines \(AB\) and \(CD\). Curves may take us slowly uphill or turn sharply. The use of lines allows the artist to demonstrate delicacy or force. Lines are used to create shape, pattern, texture\(+\), space, movement and optical illusion in design. A shape can be shown utilising an outline, and contour lines can indicate a three-dimensional structure. An essential feature of a line is that it indicates the edge of a two-dimensional (flat) shape or a three-dimensional form.
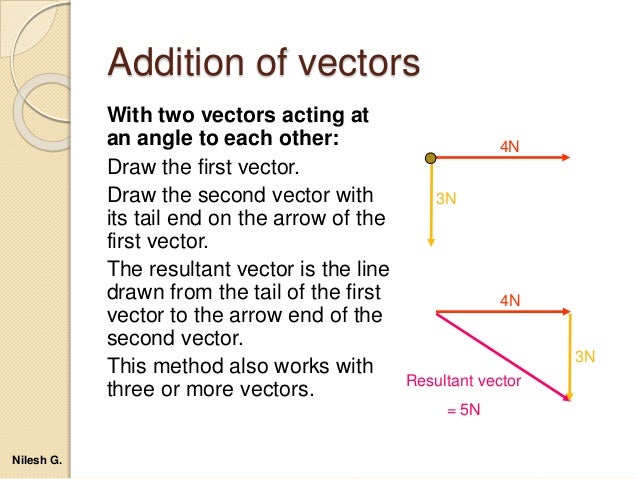
One of the most fundamental elements of art is the line. The line can be displayed by placing the arrow marks on both sides.Ī line segment can be represented by placing the bar over the letters. The line segment has two endpoints with a fixed-length The line has no endpoints with infinite length The line segment is a part of the line with a fixed-length

There are no endpoints on the line, it can extend infinitely in both directions, but the line segment has a fixed length in between two points. Line segment connects any points on the line. Learn All the Concepts of Line Segment Differences Between Line and Line Segment Television sets, bookshelves, and the globe, where latitude and longitude intersect, are all examples of perpendicular lines. Consider the following example in each of the methods.Įxample: Determine whether the four points A(1, -1, 2), B(3, -2, 5), C(1, 1, 4), and D(4, -2, 7) are coplanar.įor any four points to be coplanar, find the equation of the plane through any of the three points and see whether the fourth point satisfies it.Set squares, blackboards are also real-life examples of perpendicular lines. There are several methods to determine whether any 4 given points are coplanar.
#COPLANAR DEFINITION GEOMETRY HOW TO#
How to Determine Whether Given 4 Points are Coplanar? For example, in the above figure, A, B, E, and F are non-coplanar points. But four or more points are non-coplanar if they don't lie on a plane. Two points are never non-coplanar and three points are also never non-coplanar. Non Coplanar Points Definition in Geometry If any 3 points are taken at a time, a plane can pass through all those 3 points, and hence they are coplanar.But each of F and E are NOT coplanar with A, B, C, and D.Here are some coplanar points examples from the above figure: Remember that given any two points are always coplanar and given any three points are always coplanar. So we define the coplanar points and non-coplanar points as follows with respect to the following example:įour or more points that lie on the same plane are known as coplanar points.

But four or more points in 3D may not be coplanar. In the same way, three points in 3D can always pass through a plane and hence any 3 points are always coplanar. We know that two points in 2D can always pass through a line and hence any two points are collinear. The points that lie on the same plane are called coplanar points and hence the points that do NOT lie on the same plane are called non-coplanar points.


 0 kommentar(er)
0 kommentar(er)
